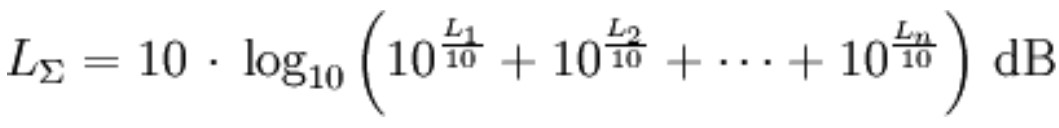Knowledge Base
Basic Acoustic Quantities
- Silence
- Audible sound
- Atmospheric pressure
- Sound pressure
The instantaneous sound pressure is the deviation from the local ambient pressure p0 caused by a sound wave at a given location and given instant in time.
The effective sound pressure is the root mean square of the instantaneous sound pressure over a given interval of time (or space).
Total pressure ptotal is given by:
ptotal = p0 + posc
where:
p0 = local ambient atmospheric (air) pressure,
posc = sound pressure deviation.
Intensity
In a sound wave, the complementary variable to sound pressure is the acoustic particle velocity. Together they determine the acoustic intensity of the wave. The local instantaneous sound intensity is the product of the sound pressure and the acoustic particle velocity
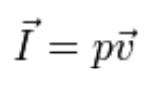
Acoustic impedance
For small amplitudes, sound pressure and particle velocity are linearly related and their ratio is the acoustic impedance. The acoustic impedance depends on both the characteristics of the wave and the transmission medium. The acoustic impedance is given by
p = vAZ
where:
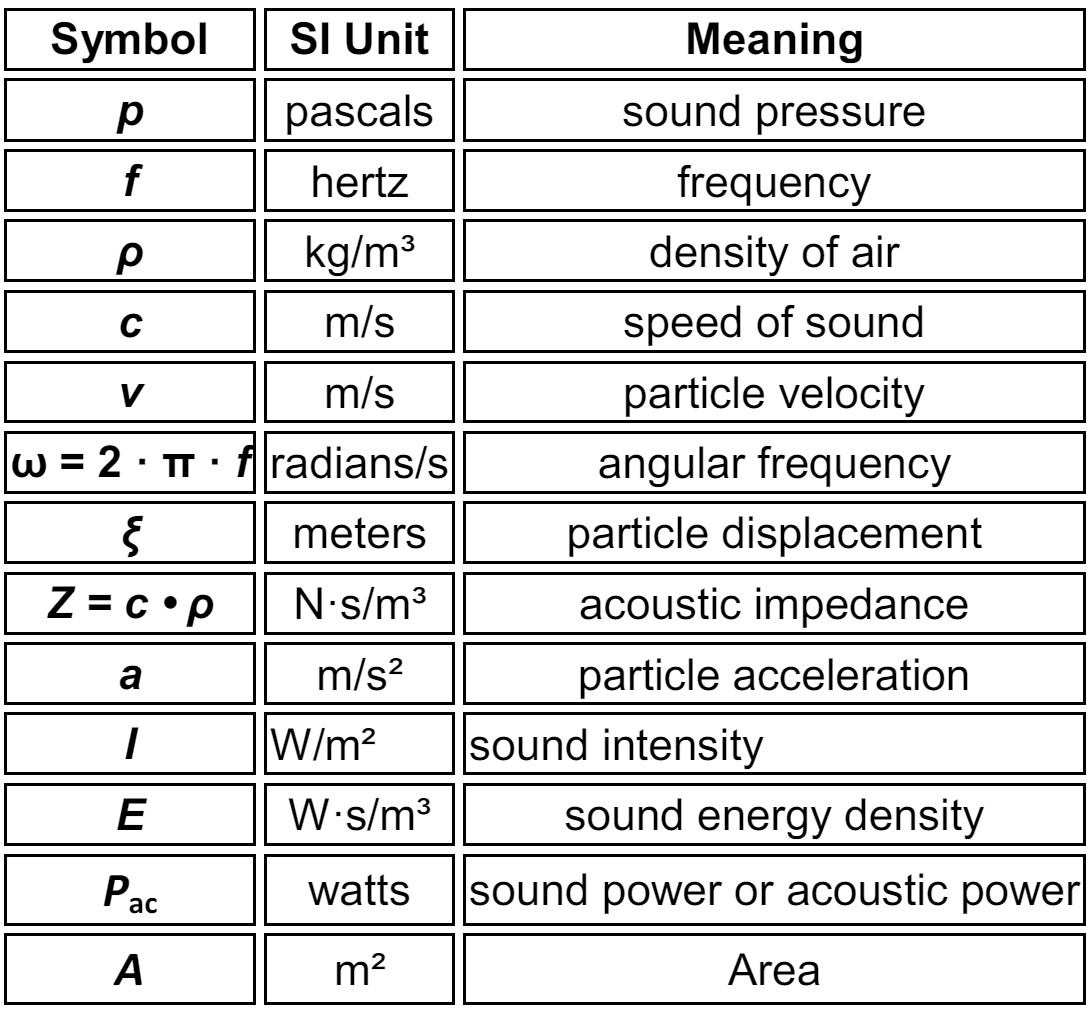
Z is acoustic impedance, sound impedance, or characteristic impedance, in Pa·s/m
v is particle velocity in m/s
I is acoustic intensity or sound intensity, in W/m2
A is surface area in m2
Particle displacement
Sound pressure p is connected to particle displacement (or particle amplitude) ξ by

Sound pressure p is
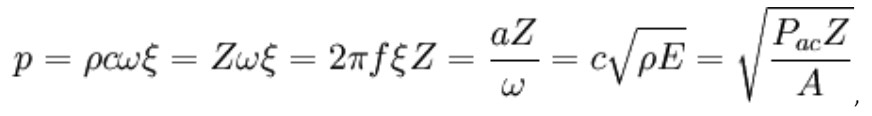
normally in units of N/m² = Pa.
where:
Distance law
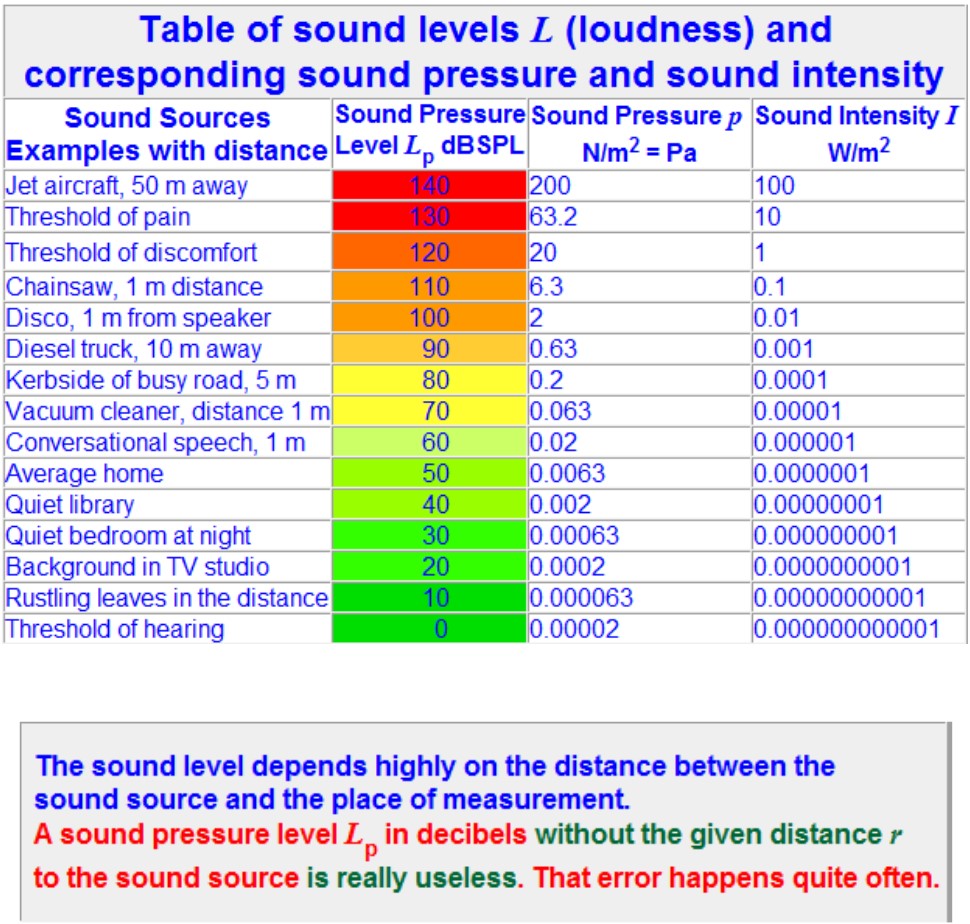
When measuring the sound created by an object, it is important to measure the distance from the object as well, since the sound pressure decreases with distance from a point source with a 1/r relationship (and not 1/r2, like sound intensity).
The distance law for the sound pressure p in 3D is inverse-proportional to the distance r of a punctual sound source.

If sound pressure is measured at a distance, one can calculate the sound pressure at another position

The assumption of 1/r² with the square is here wrong. That is only correct for sound intensity.
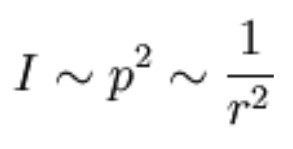
The sound pressure may vary in direction from the source, as well, so measurements at different angles may be necessary, depending on the situation. An obvious example of a source that varies in level in different directions is a bullhorn.
Note: The often used term "intensity of sound pressure" is not correct. Use "magnitude", "strength", "amplitude", or "level" instead. "Sound intensity" is sound power per unit area, while "pressure" is a measure of force per unit area. Intensity is not equivalent to pressure.
Sound pressure level
Sound pressure level (SPL) or sound level Lp is a logarithmic measure of the effective sound pressure of a sound relative to a reference value. It is measured in decibels (dB) above a standard reference level.
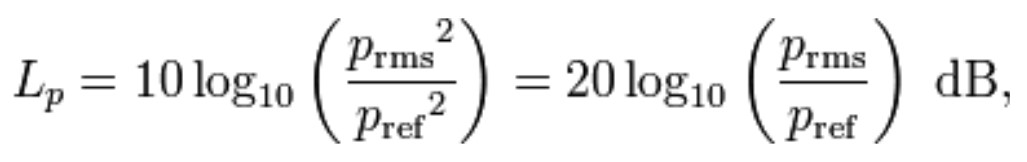
where pref is the reference sound pressure and prms is the rms sound pressure being measured
Sometimes variants are used such as dB (SPL), dB SPL, or dBSPL. These variants are not recognized as units in the SI
The unit dB (SPL) is often abbreviated to just "dB", which can give the erroneous impression that a dB is an absolute unit by itself.
The commonly used reference sound pressure in air is pref = 20 µPa (rms), which is usually considered the threshold of human hearing (roughly the sound of a mosquito flying 3 m away). When dealing with hearing, the perceived loudness of a sound correlates roughly logarithmically to its sound pressure (see Weber–Fechner law). Most measurements of audio equipment will be made relative to this level, meaning 1 pascal will equal 94 dB of sound pressure.
In other media, such as underwater, a reference level of 1 µPa is more often used.
These references are defined in ANSI S1.1-1994.
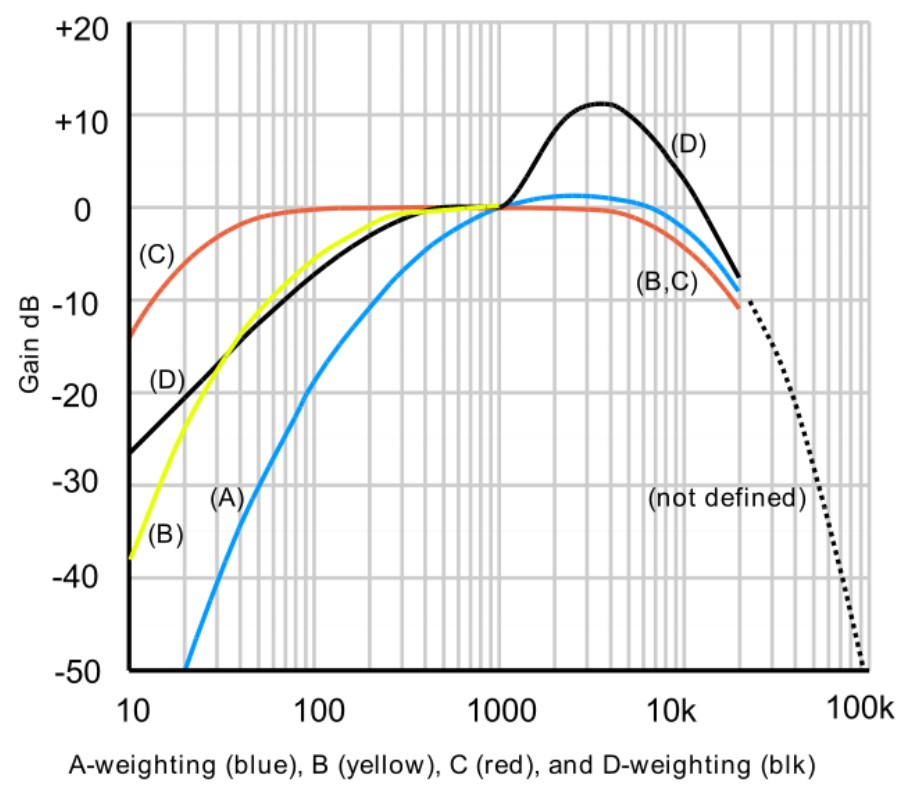
Ears detect changes in sound pressure. Human hearing does not have a flat spectral sensitivity (frequency response) relative to frequency versus amplitude. Humans do not perceive low- and high-frequency sounds as well as sounds near 2,000 Hz, as shown in the equal-loudness contour. Because the frequency response of human hearing changes with amplitude, three weightings have been established for measuring sound pressure: A, B and C. A-weighting applies to sound pressures up to 55 dB SPL, B-weighting applies to sound pressures between 55 and 85 dB SPL, and C-weighting is for measuring sound pressure above 85 dB SPL.
In order to distinguish the different sound measures a suffix is used: A-weighted sound pressure level is written either as dBA or LA. B-weighted sound pressure level is written either as dBB or LB, and C-weighted sound pressure level is written either as dBC or LC. Unweighted sound pressure level is called "linear sound pressure level" and is often written as dBL or just L. Some sound measuring instruments the use the letter "Z" as an indication of linear SPL.
Multiple sources
The formula for the sum of the sound pressure levels of n incoherent radiating sources is

From the formula of the sound pressure level we find
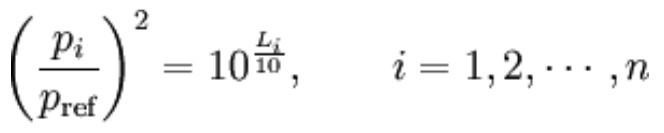
This inserted in the formula for the sound pressure level to calculate the sum level shows